Simple Transmission Networks: A Student Project

 My name is Neha Deosthali and I am a junior at the Liberal Arts and Science Academy in Austin, Texas. During the initial stages of this pandemic, I got interested in how the virus spreads through a community and wanted to learn more about the SARS-CoV-2 Virus. Thanks to Dr. Spencer Fox and Dr. Lauren Meyers, I was able to join the UT COVID Consortium where I reached out to Dr. Jennifer Johnson-Leung. Dr. Johnson-Leung has been very kind in helping me create models to answer these questions:

How does a virus spread in a small community without any countermeasures?
How many people in the population will be infected by the end of a 36 day period?
How does a virus spread in a small community with social distancing mandates in place?
How does a virus spread in a community with people wearing masks, specifically N95 masks? 

Below are the models I created to answer each of the questions respectively. The statistics such as basic reproduction number and latency period have been used from the following article in the eLife scientific journal:

    Bar-On, Yinon M, et al. “Science Forum: SARS-CoV-2 (COVID-19) by the Numbers.” ELife, ELife Sciences Publications, Ltd, 31 Mar. 2020, elifesciences.org/articles/57309.

Without Any Countermeasures:

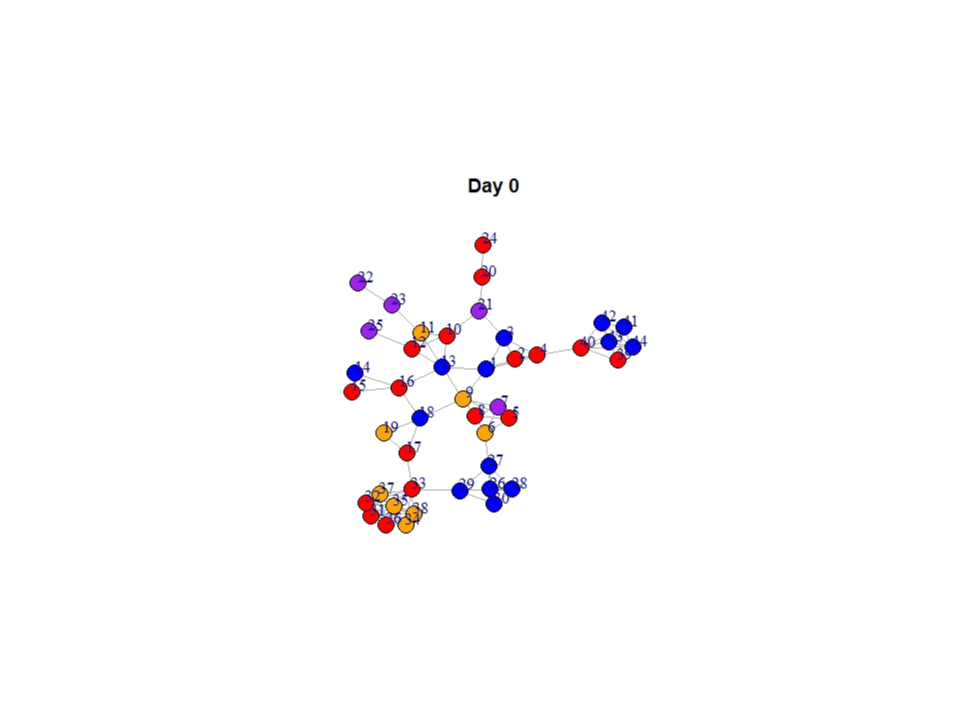
First individual infected= individual 29

First individual infected= individual 31

    *I have created two different models to explore how the spread in a population can change if the infection starts in different groups of people

    These models show the spread of COVID-19 in a small community without any countermeasures such as social distancing and mask wearing. The data used to create these models also ignores the effects of superspreaders. When a circle becomes white they are infected. The different colors represent different ages.The orange circles are 3-6 years old, blue are 10-18 year olds, red are 20-55 years, and purple are 60-80 year olds. It is assumed that age doesn’t affect the transmission rate, but it does affect the recovery rate which is why age is included in the models.

    The basic reproduction number, R0,suggests that each infected individual directly infects around 2-4 people without any countermeasures. After an individual is infected, there is a latency period of about 3 days before that individual can transmit the disease to anyone else. 

Another thing included in the model, following the 3 day latency period, is a 4 day period of maximum infectiousness, although some are infectious longer. Finally, there is a recovery period of generally 14 days or longer (Bar-On). 

    This model shows that without proper countermeasures, the virus can spread rapidly throughout a population. In both scenarios, within the span of 36 days, around 38 out of 44 individuals have been infected and only 9 recovered. This means that 86% of the population was infected in 36 days. Additionally, it can be seen that the virus infects all individuals regardless of age, although younger and older people may take longer to recover.

With Social Distancing:

The next two models show the spread of COVID-19 in a small community with physical distancing but no mask wearing.

First individual infected= individual 29

First individual infected= individual 31

The basic reproduction number, R0,suggests that each infected individual directly infects around 0.1 people per day. Similar to the ‘Without any Countermeasures’ models, after an individual is infected, there is a latency period of about 3 days followed by a 4 day maximum infectioness period and 14 day or longer recovery period (Bar-On).

    This model clearly shows that solely physical distancing can play a big role in inhibiting the spread of COVID-19. At the end of the 36 day period, only 13 out of 44 individuals had been infected. This means that physical distancing alone lowered the percentage of the infected population from 86% to 29%. The next two models will show how mask wearing can further reduce the spread of SARS-CoV-2. 

With N95 Masks (no physical distancing) 

The next two models show the spread of COVID-19 in a small community with every person wearing N95 masks.

First individual infected= individual 29

First individual infected= individual 31

These two models show the spread of COVID-19 in a small community with every person wearing N95 masks. N95 masks are able to filter out more than 95% of all particles that are at least 3 microns in diameter, and SARS-CoV-2 virus in 0.1 microns in diameter (Bar-On).

    This model clearly shows that wearing some of the best masks can substantially lower the spread of COVID-19 in a population. At the end of the 36 day period, only 6 out of 44 people were infected. This means with N95 masks, only 13% of the population is infected. That is 73% lower than in a community with no precautions and 16% lower than with only physical distancing. It is clear that wearing a mask lowers the spread of the virus, but the infection rate can be lowered much more with both physical distancing and masks. Slowing the spread of the virus would also give time for scientists, political figures, and a community in general to act upon the pandemic. In conclusion, a low infection rate is only possible when everyone wears masks and limits social gatherings. 

What I have learned from it:

Personally, working on this project was a great experience. As a high school student, modeling is something I never thought of doing before. This experience taught me a lot about the learning process. With the help of Dr. Jennifer Johnson-Leung, I was able to learn a new skill all on my own, work through the failures, problem solve, and break my comfort zone to create something new.

Important Takeaways:

From these models take into consideration how much physical distancing and mask wearing can slow and reduce the spread of COVID-19. The virus doesn’t have an age or gender preference, though people with weaker immune systems may recover more slowly. Overall, lowering the number of interactions is the best way to stump the spread of the virus. It is important to follow protocols given by the CDC and WHO in order to keep the spread of the virus under control.